A multi-dimensional non-homogeneous Markov chain of order K to jointly study multi-pollutant exceedances

Link to full Article (pending revisions)

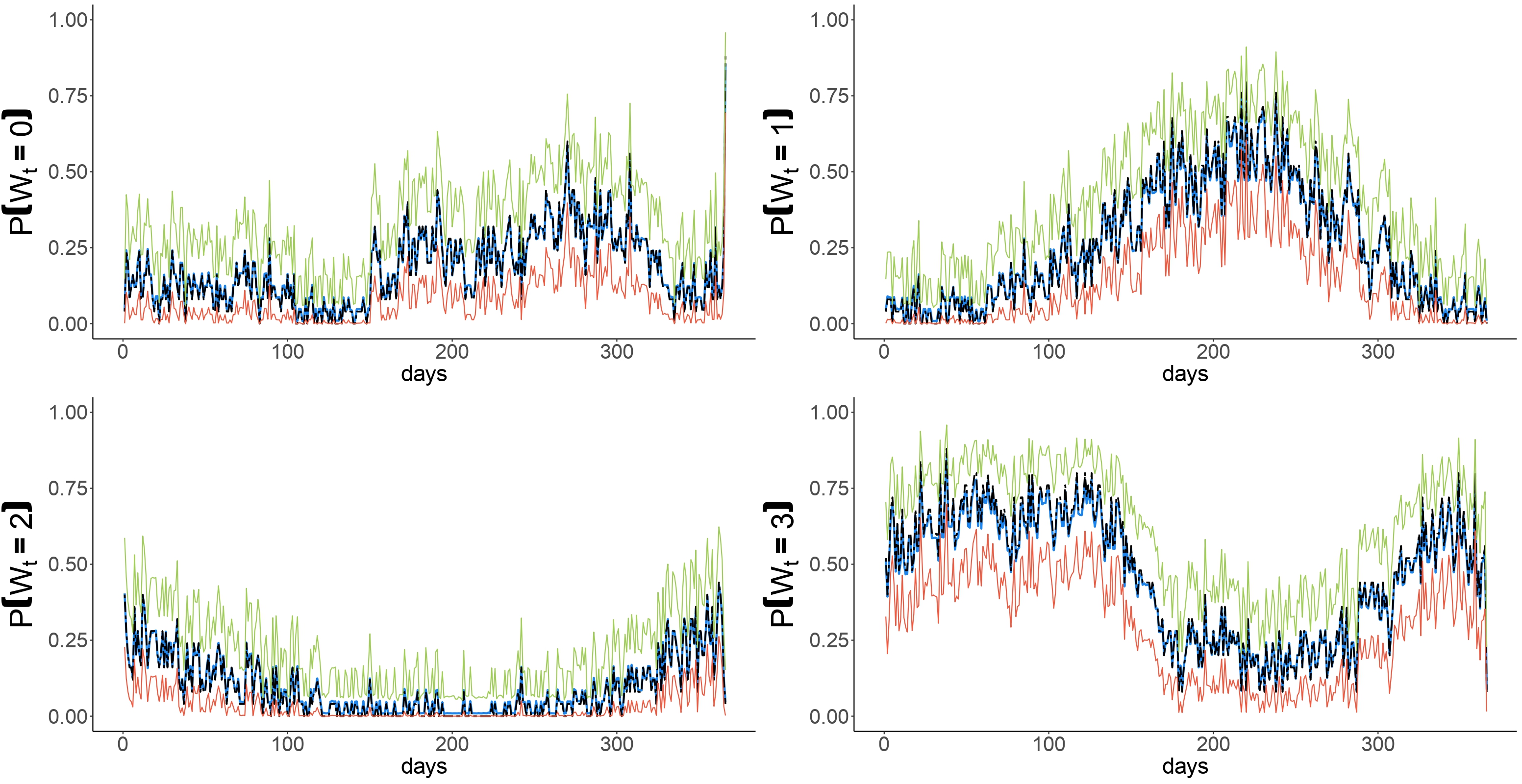
In this work we consider a multivariate non-homogeneous Markov chain of order K > 0 to study the occurrences of exceedances of environmental thresholds. In the model, d > 0 pollutants may be followed and according to their respective environmental thresholds a daily measurement may be considered an exceedance or not. The parameters of the model are the order of the chain, and its initial and transition distributions. These parameters are estimated under the Bayesian point of view. The estimated order is the value that maximises its posterior distribution. In the case of the initial and transition probabilities, the estimation is made through samples generated using their respective posterior distributions. Once these parameters are obtained, we may estimate the probability of having no, one or more pollutants exceeding the associated environmental thresholds. This is made using the Markov property as well as a recurrence formula. Results are applied to the case where d = 2 which will correspond to ozone and particulate matter with diameter smaller than 10 microns (PM10) measurements obtained from the Mexico City monitoring network.